First , three major broken power consumption doctrines According to formula (1), the work required to break the ore with a weight of Q can be listed as follows: In the middle Let D 0 be the diameter of the ore, D p be the diameter of the broken product, and integrate the above formula within the limits of D p and D 0 to obtain: i in the formula is the crush ratio. [next] Use this truth to find the average diameter in the Rettinger doctrine, you can write Equations (7) and (8) are both harmonic mean methods. Both B. Gil Pitchev and F. Kick believe that the work done by the external force of the broken object is completely used to deform the object, and the deformation energy can be stored to the limit and the object is destroyed. Based on this physical basis, the theory of crushing power consumption can be described as: the geometrically similar materials of the same type are broken into products of the same shape, and the required work is proportional to their volume or weight. The content of this doctrine can be expressed by the following formula. [next] In the middle Integrate the above formula within the limits of the ore diameter (D 0 ) and the diameter of the fractured product (D p ): When applying equation (14), the calculation of the average diameter of the ore and product is also determined. According to this theory, the power consumption is a function of the diameter of the ore, and the form of the function is lgD. Therefore, the formula for calculating the average diameter derived by the former method is: Both formulas are weighted geometric averaging. Where F is the width, micron of the square mesh that 80% of the ore can pass; K 3 in the formula is a proportional coefficient, and other symbols are the same as the previous ones. [next] Second, the comparative power of the three power theory acts on the object, first deforming it. At a certain level, the object generates micro cracks. The energy is concentrated around the original and new micro-cracks, allowing it to expand. For brittle materials, the crack breaks at the moment the crack begins to propagate, because the energy has accumulated to the extent that it can cause cracking. After the material is broken, only part of the work done by the external force is converted into surface energy, and the rest is lost in heat energy. Therefore, the work required to destroy an object includes deformation energy and surface energy, ie The meaning of each symbol in the formula is the same as the previous one. The test results of RT Huji are summarized as shown below. In the same way as the industrial method, each segment of the fracture ratio of 10 is broken and the net power consumption of each segment is obtained. From the 2nd to the 5th paragraphs, it is in line with the list theory, but from 100 microns to less than 10 microns, the data obtained by the charter theory is too small, and it is more reasonable to use the Rettinger theory. The above is not accurate in the case of Gil Pitchev, and the results of the charter theory are not reliable. Lei Tingge’s theory is too far. The doctrines are similar to the actual situation in the narrower granularity range that suits it, with little error. However, in the very fine range, even the Rettinger theory does not conform to the actual situation. Therefore, Wi1=19.2 kW.hour/short ton The required power of this mill is = 16.78 × 8.3 × 1.34 = 186 horsepower (Note: 1 kW = 1.34 inch horsepower). The crushed ore material is discharged from the discharge port of width a. It is assumed that these product blocks are equally large spheres of diameter a, and n ' pieces are arranged in a row along the length of the ground, and their volume is for: Since V 2 is the volume of the mined material that has been broken, no longer consumes energy, only the volume of (V 1 -V 2 ) ore requires energy to break. Applying the Gilpicev theory, the work required to break a row of diameter D feedstock into a diameter a product is For б = 1250 kg / cm 2 and limestone E = 200,000 kg / cm 2,
What are CNC Plasma Cutting Machines?
Plasma cutting is a process that cuts through electrically conductive materials by means of an accelerated jet of hot plasma. Typical materials cut with a plasma torch include steel, Stainless steel, aluminum, brass and copper, although other conductive metals may be cut as well. Plasma cutting is often used in fabrication shops, automotive repair and restoration, industrial construction, and salvage and scrapping operations. Due to the high speed and precision cuts combined with low cost, plasma cutting sees widespread use from large-scale industrial CNC applications down to small hobbyist shops. CNC cutting machines, such as CNC plasma cutting machines, utilize their intricate software systems and components to efficiently, accurately and smoothly handle plasma cutting processes.
CNC Plasma Cutting vs. Other Cutting Methods:
While all of these options remain widely used today, CNC plasma cutting machines are typically seen as a solution for manufacturers who are concerned with quickly and efficiently cutting conductive materials up to 3″ thick. The CNC plasma cutting process has superior cutting speeds, piercing speeds, and generates very precise cut qualities. More speed and higher quality also produces superior efficiency and productivity.
Cnc Plasma Cutting Machine applications:
CNC plasma cutting machine features:
10.It can cut metal plate of advertising 3D lighting letter and flute profile letter with high cutting precision.(USA power is optional)
Effective Cutting Width (X axis)
1500mm optional
Effective Cutting Length (Y axis)
3000mm optional
Feature
Dustproof design and heavy duty frame
Drive Mode
Leadshine
Rail
Taiwan inported Hiwin Square rail
Lifting body
1set
Plasma torch
1set
File transmission
USB transmission
Cutting shape
Able to program and cut the steel plate parts with any plane shapes formed by straight lines and arcs;
Software
Fastcam Software
Plasma power source
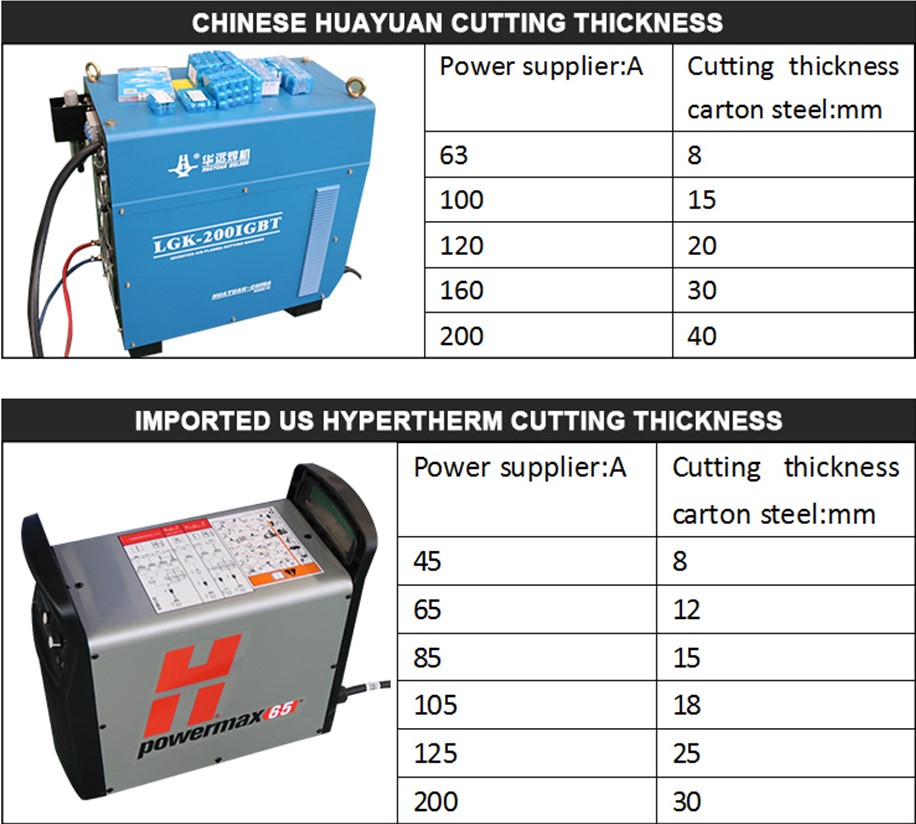
Huayuan 63A 100A 120A 160A 200A
Hypertherm 45A 65A 85A 105A 125A 200A
Cutting thickness
According to the plasma power source
Height regulating device
Arc voltage height
Cutting speed
0-10000mm/min
Cutting precision
±0.3mm
Control ccuracy
±0.01mm
Support software
Fastcam /Type3/autocad/pro/CAXA etc.
Working Temperature
-5~60°CRelative Humidity, 0-95%.
Power Supply Voltage
380V±20%(220V optional,but we need custom for you)
Packing Weight
3900x2300x1750mm
Packing Size
1500kg
Plasma Cutting Machine Plasma Cutting Machine,Cnc Plasma Cutting Machine,Plasma Flame Cutting Machine,Plasma Cutter Shandong U-May CNC Technology Co., Ltd. , https://www.fiberlasers.de
In beneficiation plant, most of the energy for crushing ore. To understand ore crushing process, evaluation of mechanical crushing efficiency, and to find a more effective method of breaking a hundred years there have been many theories about the power consumption of the crushing. Three of the most important ones are recognized by the world and will be introduced here.
As we all know, the crushing process does not happen automatically and is irreversible. It must be done by external forces to overcome the cohesion between its internal particles and to break. When an external force works on the ore to break it, the potential of the mineral material is also increased by the conversion of work. Therefore, the theory of crushing power consumption is essentially to clarify the relationship between the input work of the crushing process and the potential change of the ore before and after the crushing, so as to clarify how the input work is consumed. When crushing materials, its strength, ore size, product size and power consumption are directly easy to detect. Therefore, various power consumption theories must determine the relationship between them. This is the theory of various broken power consumption. Commonality. But because every theory of power consumption sees problems from different angles, their physical basis and derived mathematical forms are different.
1.PR Rittinger theory (1867)
When the material is broken, a new surface area is created and the surface area of ​​the product is necessarily increased compared to the surface area of ​​the original material. The particle located on the surface of the object is different from the internal particle. Since the number of particles adjacent to it is not enough to balance it, there is an unsaturated bond energy. When splitting an object, it is necessary to overcome the cohesive force between its internal particles, so that the internal particle becomes a surface particle, and the potential energy on the surface increases. Therefore, the broken object consumes a certain amount of work. According to this theory, PR Rettinger believes that the work done by the externally broken object is converted into the surface energy on the new surface area, so the work consumed by the crushing process is proportional to the new surface area. This meaning can be expressed as:
dA 1 =γdS ( 1 )
Where dA 1 - the work required to generate a new surface area dS;
The γ-proportion factor, which is the work required to generate a new surface area, can be used as the specific surface energy.
Let D be the diameter of the nugget, k 1 be the shape factor of the surface area determined by the diameter, and k 2 be the shape factor of the volume by the diameter, then k 1 D 2 is the surface area, and k 2 D 3 is the volume. It is also assumed that Q is the total weight of the crushed ore, δ is the weight of the ore per unit volume, and the number of nuggets having a diameter D in the total weight is: 



When applying the above formula, since the ore and product are mixed particles, their average particle size should be used for calculation. The method of calculating the average particle size is as follows.
Because the work consumed by the broken material is a function of the diameter of the material, for the Rettinger theory, the form of this function is f  . Let (D 0 ) average be the average diameter of the raw materials, (D 0 ) i be the diameter of the individual fractions in the raw material, and γi is the weight percentage of the individual fractions. When (D 0 ) averaging is extremely sufficient to represent the particle size of the material, the result calculated by using the prescribed function should be equal to the arithmetic mean obtained by the same function calculated by the same particle size.
. Let (D 0 ) average be the average diameter of the raw materials, (D 0 ) i be the diameter of the individual fractions in the raw material, and γi is the weight percentage of the individual fractions. When (D 0 ) averaging is extremely sufficient to represent the particle size of the material, the result calculated by using the prescribed function should be equal to the arithmetic mean obtained by the same function calculated by the same particle size. 

By the same token, the average diameter of the product in the Leitinger theory is calculated as: 
2.B. ЛИРΠИЧӘВ (1874) or F. Kick (1885) doctrine
When an external force acts on an elastic deformation of an object, its work is stored in the elastic body and becomes the deformation energy of the elastic body. Rock ore is a brittle material. Its stress and strain relationship are not strictly in accordance with Hooke's law in the elastic range, as expressed by the following formula.
б m =εE      ( 9 )
Where б—stress;
ε - strain;
E - elastic modulus;
m - an index close to 1, for granite , m = 1.13.
A brittle body such as the rock mine has an elastic limit close to the strength limit, so that the deformation energy formula in the elastic range can be approximated to the fracture state. Therefore, the brittle object of volume V is deformed in the range of external force O~б (strength limit), and the deformation energy stored therein is: 
dA 2 =KdV ( 11 )
Where dA 2 - the work required to break an object of volume dv;
K—The scale factor, which is the work required to break an object of a unit volume.
According to the method of the formula (4), and the meaning of the symbols used is the same, you can get: 



3.FC's Bond Theory (1952)
FC List is organized into the following empirical formula based on data obtained from experiments with general crushing and grinding equipment . When applying the list theory, it is best to follow his empirical formula and the meaning of the symbols specified in it, because it is more convenient, and can be compared with many studies of the list. 
P—the width of the square mesh that 80% of the product can pass, micron;
W—will be used to break the feed of the short-ton (907.18 kg) particle size F to the product with a particle size of P, kWh/short ton;
Wi — The power consumption metric, which is to break the “theoretically infinite particle size†to 80% of the work required to pass the 100 micron mesh width (or 67% through the 200 mesh mesh), kWh/short ton After establishing the above empirical formula, Bunde has made the following theoretical explanation: When crushing ore, the work of external force firstly causes the object to deform. When the local deformation exceeds the critical point, the crack is formed, and after the crack is formed, it is stored in the object. Deformation can even spread the fracture and create a section. The useful part of the input work is converted to surface energy on the new surface, and the other parts become heat loss. Therefore, the work required to break the ore should consider both deformation energy and surface energy. The deformation energy is proportional to the volume, and the surface energy is proportional to the surface area. Assuming equal consideration of these two items, the required work should be proportional to their geometric mean, ie  Proportionate. For an object of unit volume, it is
Proportionate. For an object of unit volume, it is  Proportionate. For an object of unit volume, it is proportional to.
Proportionate. For an object of unit volume, it is proportional to.
According to the explanation given by the list, it is not difficult to introduce the method of deriving the public work of the first two power doctrines: 
If you want to calculate the average particle size of the ore and product, you can use the methods of deriving public workers (7) and (15) to get:


Modern research has proved that the above-mentioned failure process, crack depth and crack expansion speed have been measured. For example, the maximum expansion speed of cracks in glass, the theoretical value is 2.0 × 10 5 cm / sec, and the observed value is 1.5 ~ 2.0 × 10 5 cm / sec. Although List cited the length of the crack to illustrate his empirical formula, it is not based on the study of the formation and expansion of cracks in modern times, but to explain the assumptions made by his empirical formula.
From the rupture process of the object mentioned above, it can be seen that each of the three theories sees a stage of the crushing process. The Gilpichev theory pays attention to the stage of deformation by external forces. The charter theory notes the formation and development of cracks. The Lei Tingge theory sees a new surface after being broken. Therefore, they all have one-sidedness, but they are not contradictory, but complement each other.
Because these three doctrines each see a stage of the fragmentation process, each theory can only be used in a certain fragmentation range to be more reliable. The literature on power consumption theory points out that the crushing ratio is not large when crushing ore, new students The surface area is not much, and the deformation can account for the main part, so the error with the Gilpicev theory is small. When grinding, the crushing ratio is large, the surface area is new, and the surface energy is the main one. Therefore, it is more suitable to use the Legending theory. The official experience of the Group is determined by testing with general crushing and grinding equipment. In the case of medium crushing ratio, it is roughly in line with it. This assertion has been confirmed by the experiment of RT Huki. 
Figure 1 Relationship between the content of crushed product and specific power consumption
I—general crushing range; II—general grinding range; III—grinding limit range
According to these verifications, when applying various power consumption theories, it is necessary to pay attention to the scope of application of each doctrine and correctly select them.
The crushing process is very complicated, and many influencing factors are not taken into consideration when establishing these theories. For example, crystal defects, cracks and joints of ore, humidity, viscosity and inhomogenesis of the ore, mutual friction and extrusion between the nuggets, etc., all affect the strength of the ore and thus affect the work required to break it. Therefore, even if the doctrines are used in a range that suits them, only approximate results can be obtained, and actual data must be used for checking. [next]
Because these theories are one-sided and similar, they are still being verified continuously and exploring new and perfect theories. Despite these shortcomings, the relationship between ore strength, grain size, product size and power consumption has been determined, reflecting the essence of the crushing process to some extent. As long as we recognize their importance and shortcomings and apply them correctly, we can provide theoretical basis and method for analyzing and studying the crushing process.
Third, the application of the power theory example : using the list of doctrine to calculate the required power of the mill.
The formulas (17) and (18) are all measurable. Regarding the power consumption indicator Wi, List has developed several assays. In order to facilitate the application of the formula, the future researchers have proposed a simpler method, as follows.
Under the same conditions, use the same mill to separately grind the same weight of standard ore (power consumption index is known, W i2 = 19.5 kW • hour / short ton), and the ore to be tested (power consumption indicator W il unknown), from The F and P values ​​found in their screening analysis curves for feeds and products are as follows:
F 1 = 960 μm of the ore to be tested P 1 = 123 μm F 2 = 1130 μm of the standard ore P 2 = 133 μm Now the mill is selected for the ore to be tested, and its treatment capacity is 8.3 short tons per hour. The particle size is F = 9500 microns,
The product particle size is P = 105 microns, and the required power of the mill is sought.
Since the work of grinding the same weight of two kinds of ore under the same conditions by the same mill should be equal, it can be listed from formula (17). 
Required power for the mill to be used 
Based on the calculated power requirements, a suitable mill can be selected from the catalogue.
Example: Using Gil Pitchev theory to analyze and study the power consumption of crusher. When applying this theory, you must master the power consumption of the crusher, the particle size analysis of the ore and product, the specific gravity and ultimate strength of the material and the elastic modulus. data. For rock ore with uneven organization, its ultimate strength and modulus of elasticity must be reliable through the average of several tests. The strength of the material measured in the material test is sometimes not the same as in the crusher. For example, the ore is subjected to splitting stress between the tooth plates of the jaw crusher, and its anti-cracking strength is about 1.2 times that of the tensile strength, which is different from the compressive strength in the material test, so it is necessary to pay attention to these conditions. In order to apply this theory more accurately. The following is an example of usage. [next]
In the study of the required power of crushing machinery, Л.Ђ 列В森 ( ЛеВеHCOH ) applied this theory to develop a theoretical formula for the power of the 颚, cone and roll machines. Although they are still to be further studied and evaluated, they are often cited in the literature. Taking the roller machine as an example, it can be seen that he applied this theory.
As shown in the figure below, the feed of diameter D is clamped between the two rolls. It is assumed that the ore nuggets are spheres of the same size, and n pieces of nuggets are closely arranged in a row along the length L of the pair of rolls. The volume of these incoming ore blocks is then 

Figure 2 The situation of the crusher crushing the ore block 
A=2.05L(D 2 -a 2 ) kg•cm Example: Using the Reinger theory to analyze the rate of new surface area generated by the ball mill grinding fine vermiculite The coefficient K 1 in the formula (3) contains the specific surface energy γ of the solid, It is an extremely unpredictable physical quantity. To date, there are only a few specific surface energy data of relatively pure substances. For example, the specific surface energy of NaCl is 0.46 × 10 -7 kW·h/m 2 , and the quartz is 0.5 × 10 -7 kW. • hour/ m2 , diamond is (1~2) × 10 -6 kW•h/ m2 . Therefore, it is difficult to estimate the value A 1 of the power consumption using data such as K 1 , which limits the application of this theory. It is usually used to qualitatively analyze the problem; or to determine A 1 and other data and then to find K 1 , but this does not calculate the true specific surface energy of the solid, because the measured A 1 contains all kinds of work. The following example shows that because the energy of the input mill is constant, the longer the grinding time, the more work is consumed and the larger the specific surface area generated. According to Reinger's theory, the work of crushing is directly proportional to the new surface area. In the case where the energy of the input mill is constant, the grinding time should be proportional to the fresh surface area. The test data confirms this because the ratio of specific surface area to honing time is almost constant.
Another cutting method utilizing CNC machines is oxy-fuel, using fuel gases and oxygen, which dates back about 100 years. Here, a stream of oxygen is blasted onto a metal heated to kindling temperature, turning it into a flowing metal oxide.
With CNC plasma cutting machines, a plasma torch blows an inert gas at high speeds out of a nozzle, through an electrical arc, which turns some of that gas to plasma.

2.START control system
3.Huayuan 120A plasma generator(Optional: Hypertherm power supply)
4.Cutting Material Collection Box with wheels,for easy take out products
5.High Quality cut performance,Fast cutting speed,high precision and low cost.
6.With firm and reasonable structure,the machine is easy to operate and durable for use.
7.The cutting incision is thin and tidily and can avoid the second processing.
8.High configurated CNC system,auto arc-striking and stable performance.
9.Working with computer and support G code and files of Uncannest software (specilized in cutting metal material) (optional Fastcam software)

Broken power theory and its application
The following table
Grinding time (hours)
Specific surface area (cm 2 /g)
Specific surface area / grinding time
13.5
13.5
20
twenty two
twenty four
26
28
30
32
34
5430
5620
7850
8350
8800
9250
9550 10250
11050
11450 11950
12700 12400
404
362
392
380
367
356
341,366
368
358374
365374